∂p ∂y = ∂q ∂x ∂ p ∂ y = ∂ q ∂ x. Here is a set of practice problems to accompany the conservative vector fields section of the line integrals chapter of the notes for paul dawkins calculus iii course at lamar university. First, find a potential function f for f and, second, calculate f(p1) − f(p0), where p1 is the endpoint of c and p0 is the starting point. We then develop several equivalent properties shared by all conservative vector fields. Use the fundamental theorem for line integrals to evaluate a line integral in a vector field.
26.2 path independence de nition suppose f : A conservative vector field has the property that its line integral is path independent; 17.3.1 types of curves and regions. Web for a conservative vector field , f →, so that ∇ f = f → for some scalar function , f, then for the smooth curve c given by , r → ( t), , a ≤ t ≤ b, (6.3.1) (6.3.1) ∫ c f → ⋅ d r → = ∫ c ∇ f ⋅ d r → = f ( r → ( b)) − f ( r → ( a)) = [ f ( r → ( t))] a b.
Depend on the specific path c c takes? Web the curl of a vector field is a vector field. Hence if cis a curve with initial point (1;0;0) and terminal point ( 2;2;3), then z c fdr = f( 2;2;1) f(1;0;0) = 1 3 1 = 2 3:
Line Integrals Conservative Vector Field (Ellipses) YouTube
Web for a conservative vector field , f →, so that ∇ f = f → for some scalar function , f, then for the smooth curve c given by , r → ( t),.
PPT VECTOR CALCULUS PowerPoint Presentation, free download ID5567505
Let’s take a look at a couple of examples. Web conservative vector fields and potential functions. We also discover show how to test whether a given vector field is conservative, and determine how to build.
SOLVED 2324 Is the vector field shown in the figure conservative
We also discover show how to test whether a given vector field is conservative, and determine how to build a potential function for a vector field known to be conservative. Similarly the other two partial.
In the second part, i have shown that ∂f_3/∂y=∂f_2/∂z. In this case, we can simplify the evaluation of \int_ {c} \vec {f}dr ∫ c f dr. The choice of path between two points does not change the value of. Gravitational and electric fields are examples of such vector fields. ∫c(x2 − zey)dx + (y3 − xzey)dy + (z4 − xey)dz ∫ c ( x 2 − z e y) d x + ( y 3 − x z e y) d y + ( z 4 − x e y) d z.
Web for certain vector fields, the amount of work required to move a particle from one point to another is dependent only on its initial and final positions, not on the path it takes. 26.2 path independence de nition suppose f : A conservative vector field has the property that its line integral is path independent;
Web In Vector Calculus, A Conservative Vector Field Is A Vector Field That Is The Gradient Of Some Function.
The 1st part is easy to show. Prove that f is conservative iff it is irrotational. Web the curl of a vector field is a vector field. We also discover show how to test whether a given vector field is conservative, and determine how to build a potential function for a vector field known to be conservative.
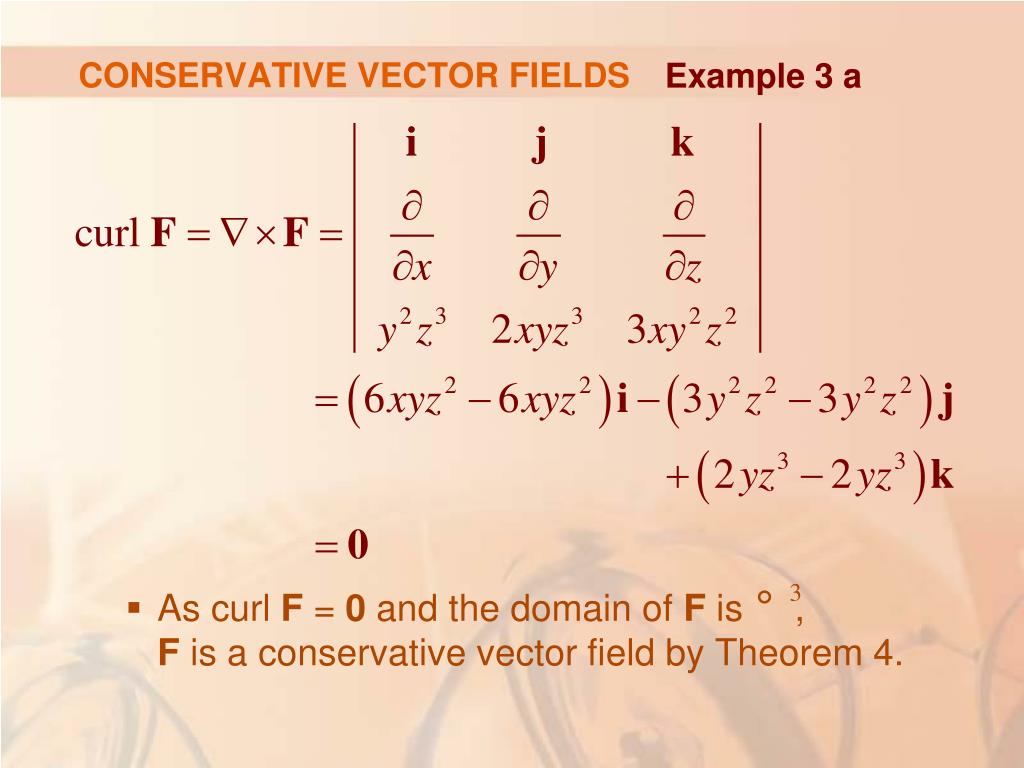
Let’s Take A Look At A Couple Of Examples.
We also discover show how to test whether a given vector field is conservative, and determine how to build a potential function for a vector field known to. 17.3.1 types of curves and regions. In this case, we can simplify the evaluation of \int_ {c} \vec {f}dr ∫ c f dr. Web a vector field f ( x, y) is called a conservative vector field if it satisfies any one of the following three properties (all of which are defined within the article):
That Is, If You Want To Compute A Line Integral (Physically Interpreted As Work) The Only Thing That Matters Is.
In fact there are fields that are not conservative but do obey \(\frac{\partial f_1}{\partial y}=\frac{\partial f_2}{\partial x}\text{.}\) we'll see one in example 2.3.14, below. As we have learned, the fundamental theorem for line integrals says that if f is conservative, then calculating ∫cf ⋅ dr has two steps: Depend on the specific path c c takes? Rn!rn is a continuous vector eld.
Use The Fundamental Theorem For Line Integrals To Evaluate A Line Integral In A Vector Field.
We then develop several equivalent properties shared by all conservative vector fields. 8.1 gradient vector fields and potentials. The aim of this chapter is to study a class of vector fields over which line integrals are independent of the particular path. The vector field →f f → is conservative.
Prove that f is conservative iff it is irrotational. Similarly the other two partial derivatives are equal. That is f is conservative then it is irrotational and if f is irrotational then it is conservative. We then develop several equivalent properties shared by all conservative vector fields. The test is followed by a procedure to find a potential function for a conservative field.